Once upon a time…
Once upon a time (about sixty years ago), all Christian parents sent their children to government schools. Then one day, the government decreed that all of the Bible reading and praying to God that were a normal part of every school day were suddenly forbidden.
A few Christians started Christian schools. A few started homeschooling their children. By the early 1980s, when James Dobson famously had Raymond Moore on Focus on the Family, and openly encouraged parents to homeschool, there were many reasons to do so; and a brave contingent of pioneering Christian parents pulled their children out of their local public schools, pulled down the blinds to protect their families from police officers who might prosecute them for this “crime”, and said, “Let’s figure this out.”
At the time, there were also voices crying out that every aspect of public education was going wrong—reading instruction, mathematics instruction, history instruction, you name it!—whichever subject the wise observer chose to inspect was shown to be filled with glaring problems, and plummeting SAT scores confirmed that the problems were not merely anecdotal, but very real.
In the Meantime…
Around the same time, a retired Air Force test pilot named John Saxon began to make waves in the math education world. In the words of a former West Point classmate:
After his retirement [from the Air Force], John taught mathematics at Rose State College in Oklahoma City. He discovered that the students were neither comprehending nor retaining what they were taught from the text books being used. At a student’s suggestion, John wrote out some problems for his class. When his students were successful in learning algebra from his writings, he decided to write a college level algebra textbook.
John was then a man with a mission, dedicated and determined to turn around the decline in math education in our country. He published two texts for the junior college level and ran successful tests with several classes of students, showing remarkable improvement in test scores and student interest. John formed his own publishing company by mortgaging his home and drawing from his savings. He authored or coauthored 13 math text books in grades K–12 and one each on calculus and physics. His books and teaching techniques have been a huge success with most teachers and students and they are lavish in their praise. Students using his books have consistently tested well above those using currently established texts. As an outsider to the math community, John fought an onslaught of criticism and opposition from what he called the “mathematics education establishment.” With a passionate belief in his cause, John spoke out with challenges to test his methods and results.
He was featured in Reader’s Digest, Time Magazine, Newsweek, USA Today, 60 Minutes, and many other publications as well as radio and television. A nationally known speaker, he travelled extensively, beating the drum for the improvement of the math skills of our youth. Currently, school districts in all 50 states and several foreign countries use his texts.
John Saxon’s Philosophy
John Saxon’s philosophy can be summed up by saying that students should understand math. He taught that math concepts should be “introduced in small units so that they may be practiced for a period of time before the next part of the same concept is introduced.” This is why his textbooks are titled, “An Incremental Development.” And he advocated that the way students came to understand math was by doing it. Again, and again, and again. In the first textbook he published, he explained more about his philosophy. He wrote:
Algebra is not difficult. Algebra is just different, and time is required in order for different things to become familiar. In this book we provide the necessary time by reviewing all concepts in every Problem Set. …
The Problem Sets contain all the review that is necessary. Your task is to work all the problems in every Problem Set. … Don’t be discouraged when you continue to make mistakes. Everyone makes these mistakes, and makes them often, and for a long period of time. A large part of learning algebra is devising defense mechanisms to protect you from yourself. If you work at it, you can find ways to prevent these mistakes. Your teacher is an expert because your teacher has made the same mistakes many times and has finally found ways to prevent them. You must do the same. …
The repetition is necessary to permit all students to master all of the concepts, and then the application must be practiced for a long time to insure retention. This practice has an element of drudgery to it, but it has been demonstrated that people who are not willing to practice fundamentals often find success elusive. Ask you favorite athletic coach for his opinion on the necessity of practicing fundamental skills.
Old-fashioned practice. Hard work.
The education establishment hated it. They would not repent of the changes they’d made to mathematics instruction in America, nor allow him to share his ideas, but boycotted his books and openly argued against his ideas in the public square. Saxon was featured in an Education Week article entitled (because, of course) ”Math’s Angry Man”:
Eventually, Saxon explains how it is that a former Air Force officer became a crusading publisher of math textbooks. When he retired from the military in 1970, Saxon had, among other things, spent five years as a test pilot, flown 55 combat missions in Korea, and taught electrical engineering at the U.S. Air Force Academy. Saxon enjoyed teaching, so he accepted a position as an algebra instructor at Oscar Rose Junior College (now Rose State College) in Midwest City, Okla. But at the end of his first semester, only 10 percent of the students had passed the final exam.
Saxon decided to change his entire approach to teaching mathematics. Instead of introducing one new concept a day, the way traditional math books do, he resolved to spend just a few minutes at the beginning of each class on new material; students would use the remainder of the period working on material they had already learned. “I finally figured out,” he says, “that you learn to work problems by working them repetitively, over a long period of time.” This concept remains the heart of the Saxon approach to math. …
Eventually, he wrote an algebra textbook for high schoolers, but the textbook publishing companies would not publish it, so he set out to prove that it really was helpful.
20 Oklahoma teachers agreed to test Saxon’s book side-by-side with another algebra textbook. Participating in the study were 1,360 9th graders, 841 of whom used the regular textbook and 519 of whom used Saxon’s. At the end of the study, the students were tested, and the results—certified by the Oklahoma Federation of Teachers—showed that the Saxon students outscored the others by more than two to one.
Armed with his test data, Saxon began seeking publicity [the author can’t conceive that Saxon wants to help teachers and students; no, he just wants fame for himself…]. He wrote a letter to conservative author and publisher William F. Buckley Jr., who was so impressed that he managed to secure a $6,500 grant to help Saxon defray his expenses. Buckley also encouraged Saxon to write an article about his math method for The National Review; Saxon wrote two of them, and they struck a chord with the Review’s conservative readers. Buckley championed Saxon in his syndicated column, writing: “He will probably figure as prominently in the history of mathematical pedagogy as Hyman Rickover in the history of the development of nuclear submarines.” …
Now, the author will finally move beyond the subtle insults and (in the pretend-unbiased voice) launch the establishment’s attacks against Saxon.
Probably the most controversial aspect of Saxon’s approach to math is its use of what he calls “gentle repetition.” New topics are introduced in bits and pieces, and old concepts are constantly reviewed. “Instead of having 20 problems illustrating a new concept,” he says, “we have 24 or 25 review problems and only four to six problems of the new kind. Certainly, four to six problems of the new kind are not enough for the students to ‘get it’ right away, but, since topics are never dropped in the Saxon books and are practiced in every subsequent problem set, by the end of the year, the students do ‘get it.’”
Critics of Saxon’s approach maintain that “gentle repetition” is nothing more than another term for “drill and practice” or “rote learning.” Hogwash, says Saxon. The naysayers, he says, “don’t realize that there is a difference between practice, which is thoughtful, considered repetition, and drill, which is blind, mindless repetition.” Saxon scoffs at the NCTM’s [The National Council of Teachers of Mathematics] Curriculum and Evaluation Standards for School Mathematics and Professional Standards for Teaching Mathematics….
“I know of no data that supports the idea that the art of problem solving can be taught,” Saxon responds. “If we are not well-grounded in facts, our abilities to think and reason are hampered.” Frank Wang, who calls the “mindless repetition” charge against Saxon’s books “an oversimplification,” says: “One of the big buzzwords now in the education world is ‘problem solving.’ Well, we say you become a good problem solver by solving problems.”
NCTM President Mary Lindquist accuses Saxon… “There’s no great revelation in what he’s doing.” She believes his books are “too prescriptive.” Saxon’s program, she adds, “certainly doesn’t go along with what research is telling us about how students learn.” …
For [Saxon], the bottom line is that his math books get results. Schools that have used his books, he claims, show that it is possible “within three years” to: increase the number of students enrolled in academic mathematics by 50 percent to 100 percent; increase college board scores in mathematics by 20 percent to 50 percent; double enrollment in both calculus and physics classes; increase enrollment in chemistry by 20 percent to 50 percent; and decrease the number of students in nonacademic math courses by more than 50 percent. To prove his point, he has a list of schools that use his books and the results they have achieved.
Window Rock High School, located on the vast Navajo Indian Reservation in northern Arizona, began using Saxon’s math books in 1985. At the time, the school’s average score on the math section of the ACT was 11.6 (out of a possible 36 points). By 1990, the average score had jumped to 18.2, and John Saxon had become a hero to the school’s 890 students. The senior class went so far as to invite Saxon to speak at its graduation ceremony, an offer the publisher gladly accepted. When he arrived, the students lined up to get his autograph, treating him more like a movie star than a publisher of textbooks.
If Saxon’s books are so good, why is it that they have been rejected by nearly half of the 22 states that adopt textbooks at the state level? …
An evaluator for the state of Virginia, for example, cited the following reasons for turning down one of Saxon’s algebra books: “The text does not adhere to the NCTM Standards…”
The article’s author goes on to defend the NCTM’s standards, by proclaiming that the standards are endorsed by the teachers unions and some other groups (I’d never heard of any of the other groups). Trust the experts!
Here are some of the standards:
“Students will … use calculators and computer software confidently and appropriately to perform mathematical tasks.”
Okay, they list other things that sound better than that, but the whole point of calculators and software to perform a mathematical task is so that the student will not have to perform the mathematical task himself. And this is one of the things that John Saxon railed against.
“Teacher performance will shift from authoritarian models based on ‘transmission of knowledge’ and ‘drill and practice’ to student-centered methods featuring ‘stimulation of learning’ and ‘active exploration.’ Teachers will help students learn how to verbalize their mathematical ideas; explore mathematical questions…; and understand that some mathematical questions have more than one right answer.”
The most fundamental characteristic of math is that it reflects the character of God (who created it to teach us about Himself), in that it is always reliable, always sure, and that the correct answer will always be the correct answer, no matter how many times you work the problem.
Clearly, Saxon and the NCTM differ in several key areas. In Saxon’s program, students don’t use calculators until they take Algebra I; the NCTM recommends the use of “appropriate calculators” as early as kindergarten. Saxon likes to think of his program as “teacher proof” because of its heavy emphasis on textbooks. The NCTM doesn’t believe that textbooks should drive instruction…
Compare the NCTM’s standards with Saxon’s instructions:
Since the learning process is long and slow, students often have difficulty understanding and retaining explanations that are clear to the teacher doing the explaining. Comprehension of abstractions occurs over a period of time and cannot be forced, even by brilliant explanations. This means that presentations by teachers who use this book should be abbreviated as much as possible because students learn by doing and learn much less when the teacher is talking. The presentation that is brief and succinct is the most effective. The new topic presented in each lesson will appear in the problem sets for the rest of the book, and the students will comprehend the concept in due time [by doing them over and over]. Most of the class period should be devoted to the homework problems, which concentrate on review…
This method of teaching is much more effective than giving in-depth explanations that are marginally comprehended and quickly forgotten. To some teachers, a quick explanation followed by a work session where help is available is not as rewarding personally as is doing an outstanding job of “teaching”. But we must remind ourselves that our job is to see that the students learn. We have no other purpose. Since the students learn by doing the problems themselves, the teacher’s job is to find some way to ensure that every student completes all the problems in every problem set.
Even with the negative framing in the Education Week article, there is story after story, statistic after statistic, of the success of the Saxon textbooks.
But the education establishment would not use his books, nor his methods (the natural, historically normal teaching methods).
In 1993, in the first edition of Physics: An Incremental Development, Teacher’s Edition, Saxon wrote an essay entitled “The Coming Disaster in Science Education in America”. He wrote:
I believe that the present disaster in science education in America will be drastically exacerbated in the next decade because of recent actions of the National Council of Teachers of Mathematics. These actions are capricious at best and approach total irresponsibility at worst. This organization has decided, with no advanced testing whatsoever, to replace preparation for calculus, physics, chemistry, and engineering with watered-down mathematics curriculum that will emphasize the teaching of probability and statistics and will encourage the replacement of the development of paper-and-pencil skills with drills on calculators and computers. This drastic shift in emphasis will leave American students bereft of the detailed knowledge of the parts that permit the whole to be comprehended.
Back to Our Brave Homeschoolers…
When our brave homeschooling parents saw what was going on with math instruction, they’d already bucked the system in the biggest way possible, so they weren’t afraid of bucking the system when it came to choosing a math book. The homeschooling parents of those days recognized a great thing for what it was, and thus, Saxon Math became the Official Math Curriculum of Homeschoolers.
(If you don’t know this history, I’m being tongue-in-cheek here with the Offical Math Curriculum of Homeschoolers. But only by a little bit. For the first twenty years or so of the modern homeschooling movement, Saxon Math was pretty much the standard curriculum homeschoolers used.)
And they all learned math happily ever after. The End.
Not the End…
But no, our story does not end there.
In the 1980s and 1990s, homeschooling was illegal in many states, or at least was treated as such. These parents used whatever leftover books they could find, which was largely Bob Jones curriculum. There was Samuel Blumenfeld’s Alpha-Phonics, Saxon Math, and Bob Jones.
But as homeschooling continued to catch on, more homeschool curriculum publishers began to offer curriculums, so that we now have a huge array of curriculums to choose from. And some things that should have been remembered were forgotten.
In 1996, John Saxon died. In 2001, his hand-chosen successor and co-author, Frank Wang, stepped down from being the CEO of Saxon Math, but stayed on as chairman of the board. However, in early 2003, Wang left the company entirely, and in 2004, the family sold Saxon Math to a big publishing company.
The details get fuzzy from there. They didn’t sell Saxon Math directly to the big education establishment textbook companies that always hated Saxon Math and had worked tirelessly for Saxon’s entire math career to stamp it out. Yet, somehow, before you knew it, Saxon Math was owned by Houghton-Mifflin.
The question of motives immediately arises. Why would Houghton-Mifflin want to purchase Saxon Math? The little company produced many millions of dollars in annual revenue, even with the boycott by the public school establishment. So even though Houghton-Mifflin already had plenty of math textbooks (which they did not discontinue or change to make more like Saxon’s books), perhaps Houghton-Mifflin wanted that little cash cow. That’s understandable.
But as soon as Houghton-Mifflin purchased Saxon Math, the Saxon Math books all suddenly needed major revisions.
As a younger homeschooling mom, when it was time for my children to start taking formal math courses, I chose Saxon based on their proven track record. But I was confused by the new vs. old editions. I have a natural inclination toward the older things, so at first I bought the old grey and blue Math 65: An Incremental Development Saxon books. But then I “learned” that the new edition was to be preferred. I didn’t know anything about John Saxon or his story; I didn’t know that entirely different authors had made all of the revisions. I trusted all of the Saxon math gurus, who uniformly said (and still say) that homeschoolers should buy the newer editions.
But today, after five years of using the newer editions, and scratching my head more and more about things that were problematic in them, it came to a crisis point for me, and I ended up opening the can of worms that led to this article. (Okay, this is the abridged version! If you want to learn more, please read the articles cited in the footnotes to this article, and compare the textbooks for yourself.)
At this moment, I have the new editions and the old editions several of the Saxon Math books, which has allowed our family to compare them side-by-side; this is our current “collection”:
John Saxon (Genuine)
Math 65: An Incremental Development
Math 76: An Incremental Development
Algebra 1/2: An Incremental Development (a.k.a. Pre-Algebra)
Algebra I: An Incremental Development
Algebra 1 1/2: An Incremental Development (a.k.a. Algebra 2)
Advanced Mathematics: An Incremental Development
Caculus with Trigonometry and Analytic Geometry
Physics: An Incremental Development
Houghton-Mifflin “Saxon”
6/5
7/6
8/7
Algebra 1: An Incremental Development (from 2003)
—
Geometry
—
—
I cannot tell you why Houghton-Mifflin purchased the Saxon company and name.
After comparing these books side-by-side, I can, however, tell you that there are many changes in the newer editions. In every case, the changes we have found are to take the original Saxon books and make them fit with the NCTM dogmas that Saxon fought against.
We have found that in the problem sets, many of the old-fashioned drill practice problems have been removed, and in their place have been added calculator problems, probability problems, ratio problems, and the exact sorts of word problems that Saxon explained were unhelpful for students.
In addition, in the Geometry book, there are outright falsehoods about Geometry being taught in the new book. (Please note that Saxon taught Geometry in his Algebra and Advanced Mathematics books, and did not put out a separate book called Geometry.) Furthermore, none of the Geometry or Algebra are really explained well at all.
For our children, discovering the actual Saxon books has been a revelation; they keep thanking me, again and again, for finding them! Not only I can see that things are taught simply and well in the original Saxon books.
As For Not Having a Teacher…
What about having a teacher?
The old Saxon textbooks are written so that you do not need a teacher.
In the midst of this Math journey, I listened to a talk by classical Mathematics instructor Arron Kau. He had a lot of great insights, but this one in particular stood out:
Reading is an important part of our curriculum in every subject, including Math class. We should give students something hard to read and interpret. Reading Math requires a different sort of discipline from reading Paradise Lost or Pride and Prejudice.
Amen!
Now, honestly, given the newer editions of Saxon Math, which seem to be written with the express intent to confuse students and leave them with the impression that they cannot understand math, I think that Kau’s idea would not work. Having a Dr. Shormann or the myriad other Saxon Teaching videos people put out there, is really necessary with those books.
But from what I can see thus far in using the actual Saxon books, as well as the success homeschoolers had with Saxon math in the 80s and 90s, I think that we should be teaching this valuable skill to our classically-taught students—how to interpret mathematical instructions, from a written text. This is how classical students studied Euclid, and Pythagoras, and Kepler, and Newton—for centuries!
To Sum
After lots and lots of prayer for wisdom, and I think the Lord helping me, I’ve made a discovery that the whole homeschooling world seems to have forgotten. The Saxon Math books are really good. But most of the books around today, that are called “Saxon Math”, actually aren’t Saxon Math books.
Please check my sources below, and share more information with me if you learn more.
And do what you will with this information I’ve uncovered. I pray it will be helpful to you!
Thanks for dropping by; please keep us in prayer!
Sources
This article was taken from The Logic of Love: Understanding 1, expected out later this year.
Article Bibliography:
https://www.west-point.org/users/usma1949/17267/, accessed 8/18/2023.
Algebra I, An Incremental Development, by John H. Saxon, Jr. Norman, OK: Grassdale Publishers, 1982.
Education Week, https://www.edweek.org/education/maths-angry-man/1993/09; accessed 8/5/2023.
Frank Wang coauthored the Saxon Calculus and Physics books with him; Saxon asked Wang to run the Saxon Math company in 1991, which he continued to do until 2001.
Advanced Mathematics, An Incremental Development, by John H. Saxon, Jr. Norman, OK: Saxon, 1990. Emphasis in the original.
http://www.educationnews.org/Gencomm/The_Coming_Disaster_in_Science_Education_in_America.htm. Screencapture from 9/2/2006 accsesed on archive.org, through the wayback machine, on 8/18/2023.
https://www.societyforclassicallearning.org/teaching-geometry-through-euclids-elements/, accessed 8/5/2023.
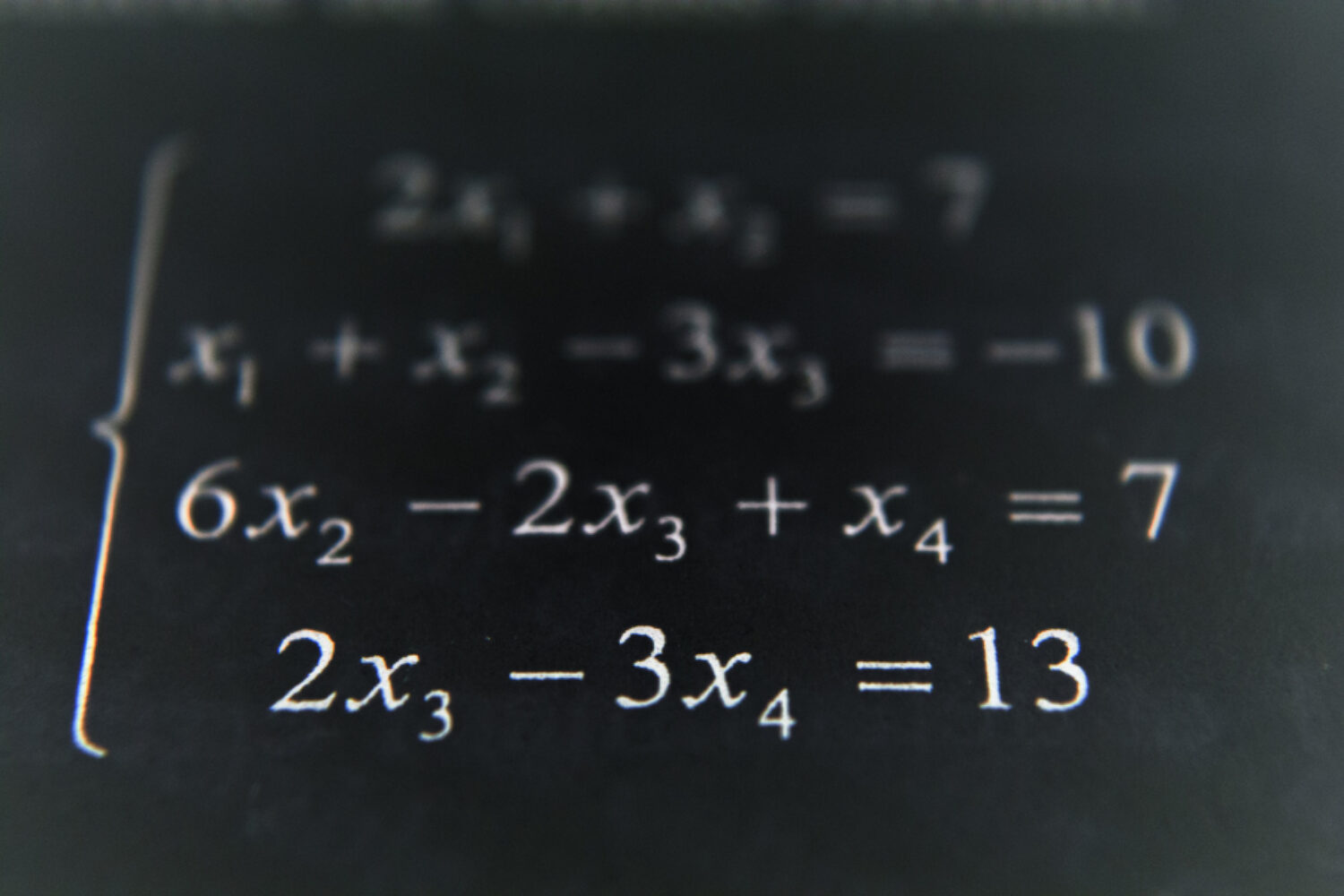
Jenn
I have used a different math curriculum for my oldest two students and have recently been contemplating switching to Saxon for the future. Since I had never attempted to purchase the Saxon books prior to now, I was surprised to find that they were owned by Houghton-Mifflin and how little information can be found on their website. As you mentioned, it seems that most families I know who use Saxon have found the only way to do it is through an online teacher explaining the topics to the students. I thought this was odd since Saxon has been such a staple in Homeschool for so long…I thought perhaps it was just that parents, educated in the public sphere, had not properly learned how to explain it. Now that I read your article, this makes so much sense. I will begin looking for the original Saxon books. Whether I use them or not (and I most likely will) I want to help preserve the originals for the future! Thank you!
Robyn Van Eck
In answer to a question about where to find the old Saxons…
Here’s the very best source – Abe Books. At Abe Books, when you perform an Advanced Search, you can select a maximum publication date. To get genuine Saxon books, I set my max publication date to 2002, before Frank Wang stepped down and the company was sold, and I was golden!
Pictures can be dicey, because sometimes the seller will used a stock image for one edition, even though they are actually holding a different edition, so to be safe I used the publication date filter at Abe.
Another great source I like is Exodus Books. When you search for Saxon books, they have a separate listing for “Old Saxon”. And they sell them really cheap! But their selection isn’t huge. Here’s that list: https://www.exodusbooks.com/saxon-math-old-versions-only/8115/
Although they’re not being printed now, the old Saxons were in lots of schools and homeschools, so plenty of used copies are floating around out there!